Synthetic Gravity

An n-body choreography is a periodic solution to the n-body problem in which all the bodies are equally spread out along a single orbit. Interesting choreographies are typically discovered using methods from topology and calculus of variations but they can be rather difficult to find in practice.

However, we can side step the problem and achieve similarly graceful behaviors with highly symmetric starting configurations. For example, if the starting positions of the points are vertex locations of the unit distance Golomb graph (shown on the left) then what we see the mesmerizing ballet on the right

All edges are length 1

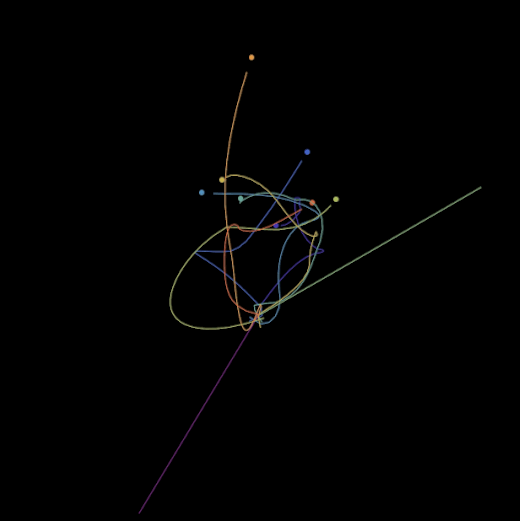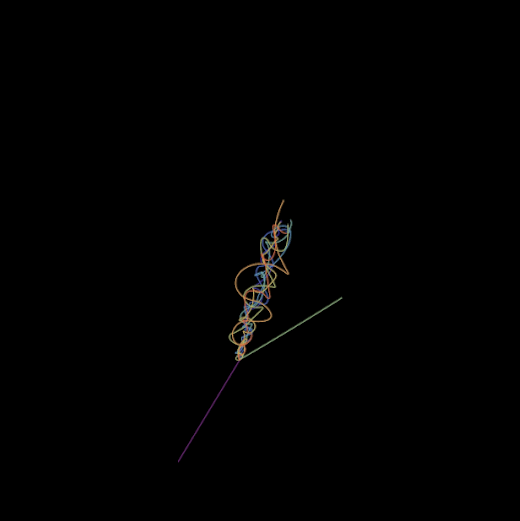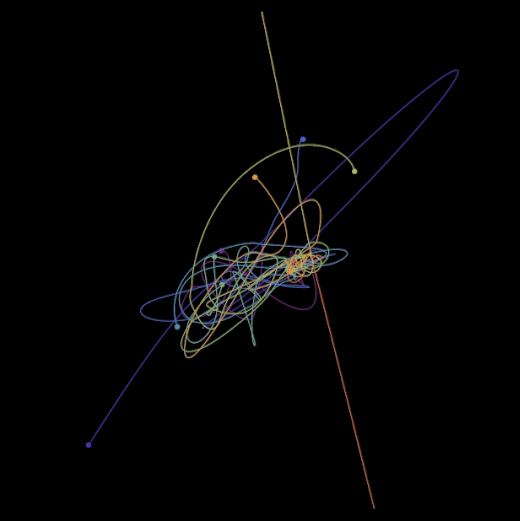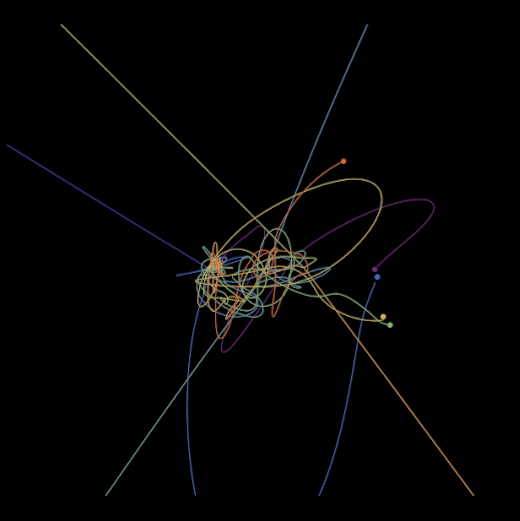
We can do the same simulation of particles in three dimensions. Starting with an anti-prism on the left the vertices weave beautifully patterned orbits if we visualize them over time.

Starting with random positions and random masses will show random behaviors that are still interesting in a modern art sense if not platonically beautiful. Get the code.